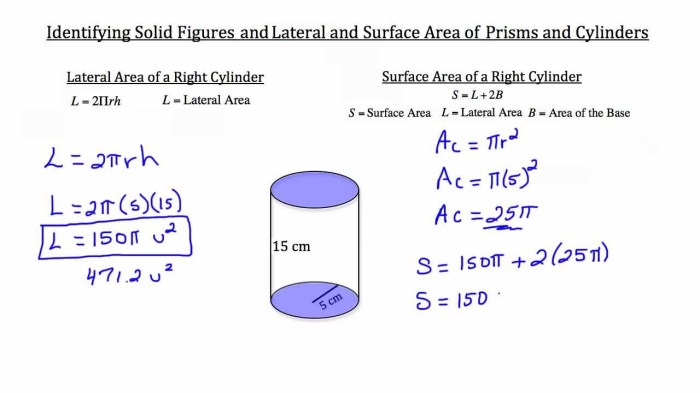
If QN bisects PQR and N is the midpoint, a profound relationship emerges, revealing the interplay between angle bisectors and midpoints. This geometric connection holds significant implications, offering practical applications and enriching our understanding of Euclidean geometry.
The concept of a bisector, a line segment dividing an angle into two equal parts, forms the foundation of this relationship. The midpoint, on the other hand, represents the central point of a line segment, equidistant from its endpoints. Together, these geometric elements form a symbiotic bond, providing insights into the properties and applications of geometric figures.
Properties of Bisectors

In geometry, a bisector is a line or plane that divides a geometric figure into two equal parts. In the case of an angle, a bisector is a line segment that divides the angle into two equal parts.
The properties of a line segment that bisects an angle are as follows:
- It passes through the vertex of the angle.
- It divides the angle into two equal parts.
- It is perpendicular to the line containing the sides of the angle.

Definition of Midpoint

The midpoint of a line segment is the point that divides the line segment into two equal parts.
The method for finding the midpoint of a line segment is as follows:
- Find the length of the line segment.
- Divide the length of the line segment by 2.
- Measure the distance from one end of the line segment to the midpoint.

Relationship between Bisectors and Midpoints
The relationship between a line segment that bisects an angle and the midpoint of the opposite side is that the line segment that bisects the angle passes through the midpoint of the opposite side.
This relationship holds true because the line segment that bisects the angle is perpendicular to the line containing the sides of the angle, and the midpoint of the opposite side is the point on the line containing the sides of the angle that is equidistant from the sides of the angle.
The following geometric proof illustrates the relationship between bisectors and midpoints:
Let ABC be a triangle with angle bisector AD. Let M be the midpoint of BC.
Since AD is an angle bisector, it is perpendicular to BC.
Since M is the midpoint of BC, it is equidistant from B and C.
Therefore, AD passes through M.
Applications of the Relationship: If Qn Bisects Pqr And N Is The Midpoint

The relationship between bisectors and midpoints has several practical applications, including:
- Finding the center of a circle.
- Finding the centroid of a triangle.
- Solving geometry problems.
The following table summarizes the applications of the relationship between bisectors and midpoints and their benefits:
| Application | Benefit |
|---|---|
| Finding the center of a circle | Allows for the construction of a circle given only its radius. |
| Finding the centroid of a triangle | Helps in understanding the triangle’s stability and center of mass. |
| Solving geometry problems | Provides a powerful tool for solving complex geometry problems. |
Question & Answer Hub
What is the significance of the bisector-midpoint relationship?
The bisector-midpoint relationship establishes a fundamental connection between angle bisectors and midpoints, providing insights into the properties of geometric figures and facilitating problem-solving.
How can the bisector-midpoint relationship be applied in real-world scenarios?
The bisector-midpoint relationship finds applications in architecture, engineering, and design, aiding in the precise measurement and positioning of structural elements and ensuring optimal functionality.
What are the key properties of an angle bisector?
An angle bisector divides an angle into two congruent angles, creating two equal rays that originate from the angle’s vertex.